使用gsl库产生高斯分布随机数

什么是GSL？

The GNU Scientific Library (GSL) is a numerical library for C and C++ programmers.

The library provides a wide range of mathematical routines such as random number generators, special functions and least-squares fitting. There are over 1000 functions in total with an extensive test suite.

The complete range of subject areas covered by the library includes,

Complex Numbers	Roots of Polynomials
Special Functions	Vectors and Matrices
Permutations	Sorting
BLAS Support	Linear Algebra
Eigensystems	Fast Fourier Transforms
Quadrature	Random Numbers
Quasi-Random Sequences	Random Distributions
Statistics	Histograms
N-Tuples	Monte Carlo Integration
Simulated Annealing	Differential Equations
Interpolation	Numerical Differentiation
Chebyshev Approximation	Series Acceleration
Discrete Hankel Transforms	Root-Finding
Minimization	Least-Squares Fitting
Physical Constants	IEEE Floating-Point
Discrete Wavelet Transforms	Basis splines
Running Statistics	Sparse Matrices and Linear Algebra

以上摘自http://www.gnu.org/software/gsl/

可见里面还是有不少干货的。

我们知道计算机产生的随机数都是伪随机数，需要一个种子，种子不同，产生的随机数序列才不同，这个种子可以是时间，因为系统的时间一直都在变。通常，我们用c标准库里的函数rand产生的随机数是均匀分布的，那么要产生高斯分布的（钟形）随机数就需要一个算法，在GSL库中实现了两个这样的算法。

这里解读其随机数产生的实现，我使用的源码是gsl-2.4.tar.gz，gsl_ran_gaussian函数就是产生高斯分布随机数。

其代码在gsl-2.4/randist/gauss.c中

double
gsl_ran_gaussian (const gsl_rng * r, const double sigma)
{
  double x, y, r2;

  do
    {
      /* choose x,y in uniform square (-1,-1) to (+1,+1) */
      x = -1 + 2 * gsl_rng_uniform_pos (r);
      y = -1 + 2 * gsl_rng_uniform_pos (r);

      /* see if it is in the unit circle */
      r2 = x * x + y * y;
    }
  while (r2 > 1.0 || r2 == 0);

  /* Box-Muller transform */
  return sigma * y * sqrt (-2.0 * log (r2) / r2);
}

这是Box-Muller方法实现的，在Knuth的The Art Of Computer Programming Vol.2, 3rd edition里有讲到。

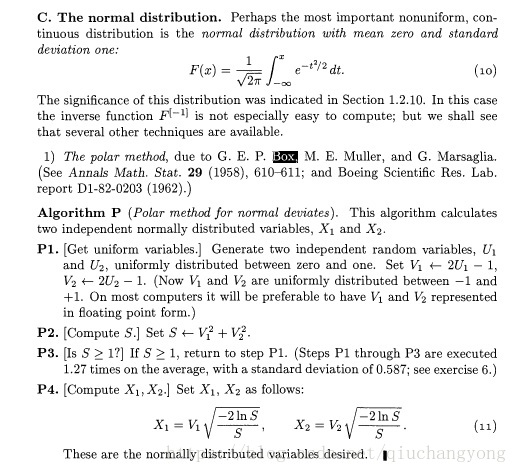
另一个方法是Kinderman-Monahan方法，有相关论文，这里不讲。

上面那个函数中的gsl_rng_uniform_pos就是产生均匀分布的随机数，范围是(0, 1)

其实现在gsl-2.4/rng/gsl_rng.h中，

INLINE_FUN double
gsl_rng_uniform_pos (const gsl_rng * r)
{
  double x ;
  do
    {
      x = (r->type->get_double) (r->state) ;
    }
  while (x == 0) ;

  return x ;
}
如果要在深挖下去，就到了gsl_rng_type *gsl_rng_mt19937

get_double就是gsl_rng_mt19937的成员函数，具体实现在gsl-2.4/rng/mt.c中。

它也可以用rand() / (RAND_MAX + 1.0)代替，那样就要在产生随机数之前用srand设置一个随机数种子。

附：

#define RAND_MAX 0x7fff

static double
mt_get_double (void * vstate)
{
  return mt_get (vstate) / 4294967296.0 ;
}
可以看出还是有点不同，后者除以(0xFFFFFFFF+1)