hdoj1710Binary Tree Traversals

最新推荐文章于 2021-09-21 17:05:11 发布

铁兵

最新推荐文章于 2021-09-21 17:05:11 发布

阅读量1.9k

点赞数 2

分类专栏： acm 文章标签： tree traversal integer input each output

本文链接：https://blog.youkuaiyun.com/teibin/article/details/7583600

版权

acm 专栏收录该内容

8 篇文章

订阅专栏

Binary Tree Traversals

Time Limit: 1000/1000 MS (Java/Others) Memory Limit: 32768/32768 K (Java/Others)
Total Submission(s): 1406 Accepted Submission(s): 655

Problem Description

A binary tree is a finite set of vertices that is either empty or consists of a root r and two disjoint binary trees called the left and right subtrees. There are three most important ways in which the vertices of a binary tree can be systematically traversed or ordered. They are preorder, inorder and postorder. Let T be a binary tree with root r and subtrees T1,T2.

In a preorder traversal of the vertices of T, we visit the root r followed by visiting the vertices of T1 in preorder, then the vertices of T2 in preorder.

In an inorder traversal of the vertices of T, we visit the vertices of T1 in inorder, then the root r, followed by the vertices of T2 in inorder.

In a postorder traversal of the vertices of T, we visit the vertices of T1 in postorder, then the vertices of T2 in postorder and finally we visit r.

Now you are given the preorder sequence and inorder sequence of a certain binary tree. Try to find out its postorder sequence.

Input

The input contains several test cases. The first line of each test case contains a single integer n (1<=n<=1000), the number of vertices of the binary tree. Followed by two lines, respectively indicating the preorder sequence and inorder sequence. You can assume they are always correspond to a exclusive binary tree.

Output

For each test case print a single line specifying the corresponding postorder sequence.

Sample Input

  
  
   
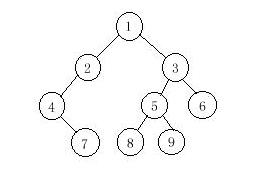
   9
1 2 4 7 3 5 8 9 6
4 7 2 1 8 5 9 3 6

Sample Output

  
  
   
   7 4 2 8 9 5 6 3 1

Source

HDU 2007-Spring Programming Contest

Recommend

lcy

本质就是知道二叉树的前序和中序求后序

#include <iostream>
#include <algorithm>

using namespace std;
int preorder[1005];
int inorder[1005];

void dfs(int pre,int in,int size,bool flag)
{
    int i;
    if(size==1) //如果有左子树或右子树，就直接输出
    {
        printf("%d ",preorder[pre]);
        return ;
    }
    if(size<=0) //没有左子树或右子树，则返回上层，遍历右子树或者根
        return ;
    for(i=0;preorder[pre]!=inorder[in+i];i++);//查找根节点在中序中的位置
    dfs(pre+1,in,i,false);              //左子树的遍历
    dfs(pre+i+1,in+i+1,size-i-1,false); //右子树的遍历
    if(flag)                           //输出根节点
        printf("%d\n",preorder[pre]); //整个树的根节点
    else
        printf("%d ",preorder[pre]);   //一般的根节点
}

int main()
{
    int n,i;
    while(cin>>n)
    {
        for(i=1;i<=n;i++)
            scanf("%d",&preorder[i]);
        for(i=1;i<=n;i++)
            scanf("%d",&inorder[i]);
        dfs(1,1,n,true);
    }
    return 0;
}