欧拉计划问题二十四matlab实现

Problem 24 ：Lexicographic permutations

A permutation is an ordered arrangement of objects. For example, 3124 is one possible permutation of the digits 1, 2, 3 and 4. If all of the permutations are listed numerically or alphabetically, we call it lexicographic order. The lexicographic permutations of 0, 1 and 2 are:

                                               012   021   102   120   201   210

What is the millionth lexicographic permutation of the digits 0, 1, 2, 3, 4, 5, 6, 7, 8 and 9?

思路 ：

整个解题的思路就是这个公式，这就是一个全排列的问题，树状图可以很好的描述这个过程，就拿题中那个举例子：

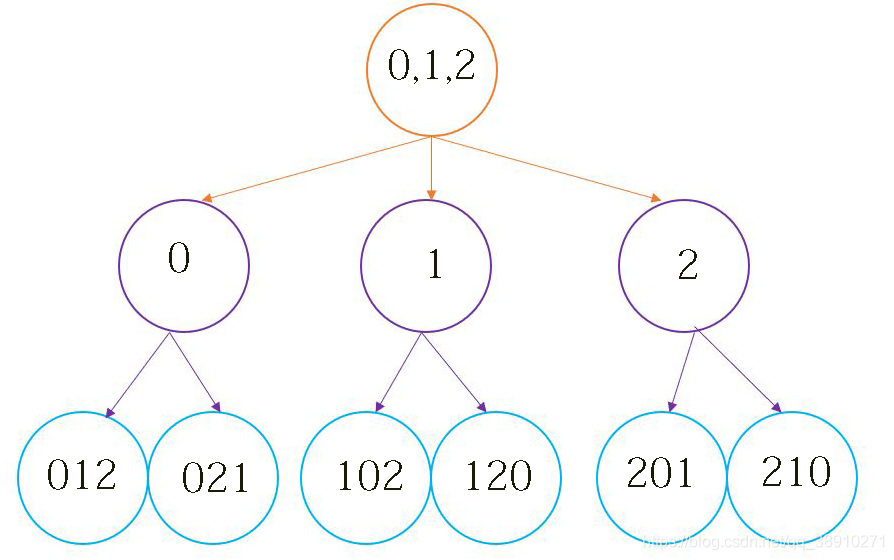
总的排列方式

 

好像一下子回到了高中，数学老师在讲台上讲排列组合还历历在目，哈哈哈！

我的思路就是给写一个for回圈，从最小的数开始----0123456789，最大的数-----9876543210结束，如果排列数满足条件，

怎么设置是否满足条件呢？我的灵感是从犹太人抄写圣经检查误差的方法得来的，这是以前一本书中的读到的（有兴趣的可以去了解一下，犹太人如何对圣经抄写本查错）所以说多读读书没错，三毛说过，你读过的书不是过眼云烟，而是潜藏在你的气质中，谈吐中，在胸襟的无涯中！

言归正传，若满足条件，就累加一次，若累加达到100万，则跳出循环，最后一个数即为结果！然而这样做起来算得太慢了，有点像电动机过载，小马拉大车，效率太低了。我觉得是范围界定得太大了，于是我便考虑缩小范围，具体的方法是这样的。

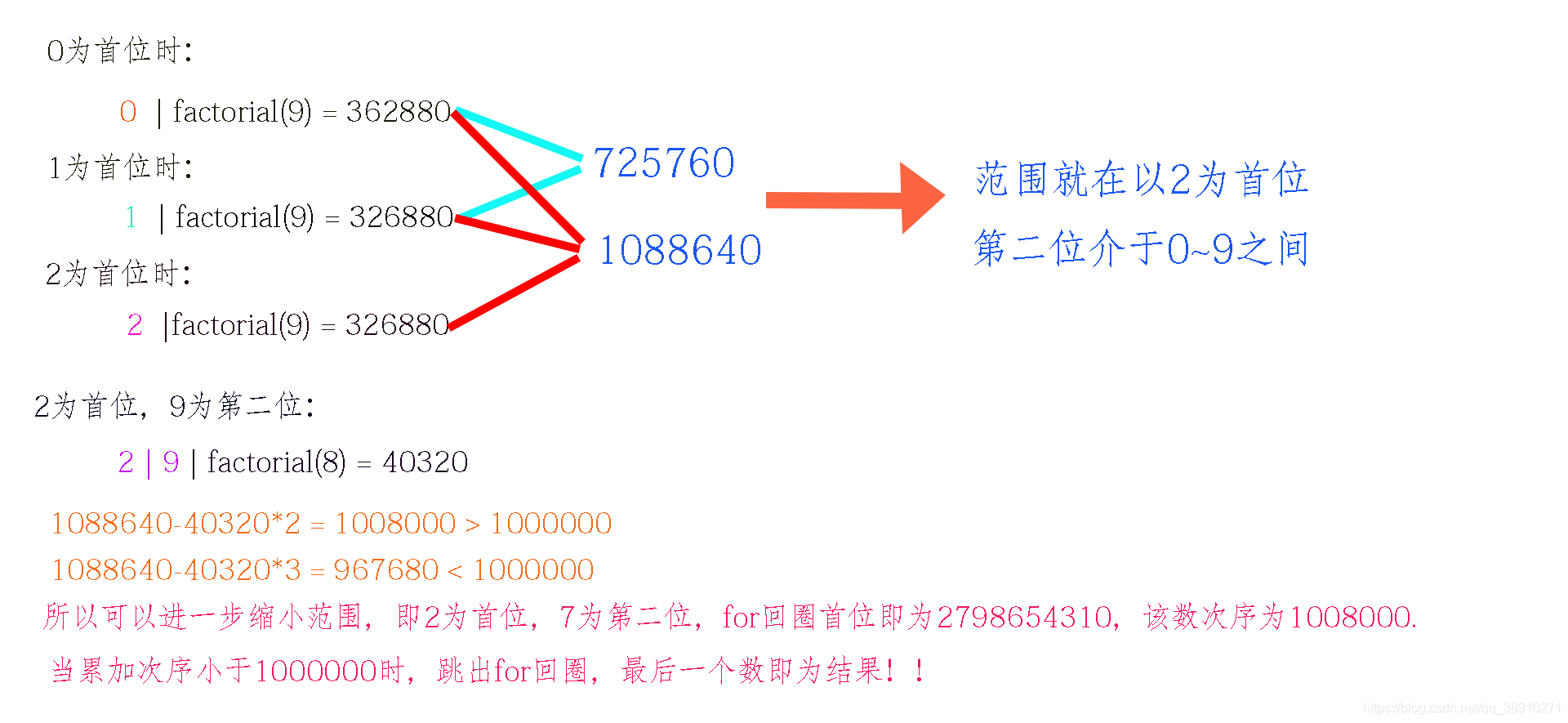
这个图就是循环初值为什么设成代码中那样的原因！

代码 ：

clear,clc
tic
k = 1008000;
A = '0123456789';
for i = 2798654310:-1:0123456789
    B = num2str(i);
    len = length(B);
    if len < length(A)
        for i = 1:len
            C(i+1) = char(B(i));
        end
        C(1) = '0';
        len = len + 1;
    else
        for i = 1:len
            C(i) = char(B(i));
        end
    end
    c = sort(C);
    if c == A
        disp(C);
        k = k - 1;
    end
    if k < 1000000
        break
    end
end
toc

结果 ：2783915460

运行出来是这样的：

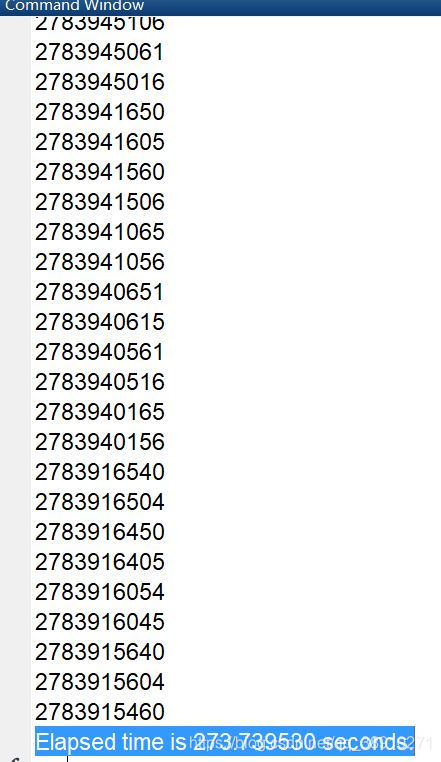
希望大家多多交流！