数据结构【树和二叉树】

于 2023-04-09 00:26:04 发布
阅读量129 收藏 1
点赞数 1
文章标签： 笔记 数据结构 c语言
版权声明：本文为博主原创文章，遵循 CC 4.0 BY-SA 版权协议，转载请附上原文出处链接和本声明。
本文链接：https://blog.youkuaiyun.com/hshsbabba/article/details/130002602
版权

目录

一.树

1.树的基本概念

2.树与非树

 3.树的相关概念

4.树的结构

 二.二叉树

1.二叉树的基本概念

2.二叉树的性质

三.二叉树的链式存储

1.存储结构

 2.二叉树的基础操作

①.二叉树的建立

②.前序遍历

③中序遍历

④.后序遍历

⑤.二叉树的结点数

⑥.二叉树的叶结点数

⑦.二叉树的第K层结点数

⑧.查找树为X的结点

⑨二叉树的层序遍历

 ⑩.判断完全二叉树

 ⑪.二叉树的销毁

四.完整代码 

1.queue.h

2.queue.c

3.tree.c

一.树

1.树的基本概念

树是一种非线性的数据结构，它是由n（n>=0）个有限结点组成一个具有层次关系的集合。

其中，A叫做根节点。 

2.树与非树

 

 

上面这三种情况都不是树。

需要满足以下情况：

①.树的子树之间是不相交的；

②.除了根结点外，每个结点有且仅有一个父结点；

③.一棵N个结点的树有N-1条边 

 3.树的相关概念

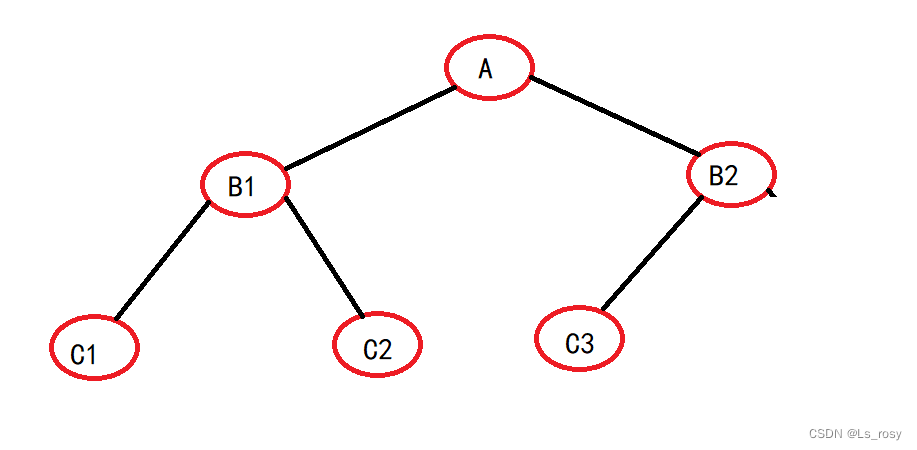
节点的度：一个节点含有的子树的个数称为该节点的度； 如上图：A的度为3
叶节点或终端节点：度为0的节点称为叶节点； 如上图：C1,C2,C3,C4,C5,C6为叶节点
非终端节点或分支节点：度不为0的节点； 如上图：A,B1,B2,B3为分支节点
双亲节点或父节点：若一个节点含有子节点，则这个节点称为其子节点的父节点； 如上图：A是B1,B2,B3的父节点
孩子节点或子节点：一个节点含有的子树的根节点称为该节点的子节点； 如上图：B1,B2,B3是A的孩子节点
兄弟节点：具有相同父节点的节点互称为兄弟节点； 如上图：B1,B2,B3是兄弟节点
树的度：一棵树中，最大的节点的度称为树的度； 如上图：树的度为3
节点的层次：从根开始定义起，根为第1层，根的子节点为第2层，以此类推；
树的高度或深度：树中节点的最大层次； 如上图：树的高度为3
堂兄弟节点：双亲在同一层的节点互为堂兄弟；如上图：C1,C3互为堂兄弟节点
节点的祖先：从根到该节点所经分支上的所有节点；如上图：A是所有节点的祖先
子孙：以某节点为根的子树中任一节点都称为该节点的子孙。如上图：所有节点都是A的子孙
森林：由m（m>0）棵互不相交的树的集合称为森林；

4.树的结构

树的一个结点中，不仅有数据，还得有一个标记来指向它的下一个结点。这个标记就是指针。

一棵树，横着看，有他的兄弟们，竖着看，有它的孩子。所以我们用一个指针指向它的第一个孩子，用另一个指针指向它的兄弟，所有结点就被串联起来了。

typedef int datatype;
struct Node
{
 datatype val;
 struct Node* child; // 第一个孩子结点
 struct Node* brother; // 指向其下一个兄弟结点
}

 二.二叉树

1.二叉树的基本概念

二叉树是指树中结点的度不大于2的有序树

而二叉树又分完全二叉树和满二叉树

1.满二叉树：一个二叉树，如果每一个层的结点数都达到最大值，则这个二叉树就是满二叉树。
，则它就是满二叉树。
2. 完全二叉树： 完全二叉树是效率很高的数据结构，完全二叉树是由满二叉树而引出来的。对于深度为K的，有n个结点的二叉树，当且仅当其每一个结点都与深度为K的满二叉树中编号从1至n的结点一一对应时称之为完全二叉树。 要注意的是满二叉树是一种特殊的完全二叉树。
完全二叉树：

满二叉树：

2.二叉树的性质

1. 若规定根节点的层数为1，则一棵非空二叉树的第i层上最多有2^(i-1)个结点.
2. 若规定根节点的层数为1，则深度为h的二叉树的最大结点数是 2^h - 1
3. 对任何一棵二叉树, 如果度为0其叶结点个数为x , 度为2的分支结点个数为y ,则有 x=y+1
4. 若规定根节点的层数为1，具有n个结点的满二叉树的深度，h=log(N+1) 以2为底
5. 对于具有n个结点的完全二叉树，如果按照从上至下从左至右的数组顺序对所有节点从0开始编号，则对 于序号为i的结点有：
1. 若i>0，i位置节点的双亲序号：(i-1)/2；i=0，i为根节点编号，无双亲节点
2. 若2i+1<n，左孩子序号：2i+1，2i+1>=n否则无左孩子
3. 若2i+2<n，右孩子序号：2i+2，2i+2>=n否则无右孩子

三.二叉树的链式存储

1.存储结构

typedef struct TreeNode
{
	char data;                 //数据域
	struct TreeNode* left;     //左孩子
	struct TreeNode* right;    //右孩子
}TreeNode;

 2.二叉树的基础操作

①.二叉树的建立

TreeNode* rootBinaryTreeCreate(char* arr, int n, int* i)
{
	TreeNode* node = (TreeNode*)malloc(sizeof(TreeNode) * n);
	if (arr[*i] == '#')
	{
		(*i)++;              //++和* 优先级问题
		return NULL;
	}
	
	node->data = arr[(*i)++];            // ++和* 优先级问题
	node->left = rootBinaryTreeCreate(arr, n, i);
	node->right = rootBinaryTreeCreate(arr, n, i);

	return node;

}

int main()
{
	char arr[] = "ABD##E#H##CF##G##";  // 不为 # 就进入二叉树
	int sz = strlen(arr);
	int i = 0;
	TreeNode* root=rootBinaryTreeCreate(arr,sz,&i);
}

②.前序遍历

void BinaryTreePrevOrder(TreeNode* root)
{
	if (root == NULL)
	{
		return NULL;
	}

	printf("%c ", root->data);
	BinaryTreePrevOrder(root->left);
	BinaryTreePrevOrder(root->right);
}

③中序遍历

void BinaryTreeInOrder(TreeNode* root)
{
	if (root == NULL)
	{
		return NULL;
	}

	BinaryTreeInOrder(root->left);
	printf("%c ", root->data);
	BinaryTreeInOrder(root->right);
}

④.后序遍历

void BinaryTreePostOrder(TreeNode* root)
{
	if (root == NULL)
	{
		return NULL;
	}

	BinaryTreePostOrder(root->left);
	BinaryTreePostOrder(root->right);
	printf("%c ", root->data);
}

⑤.二叉树的结点数

int BinaryTreeSize(TreeNode* root)
{
	if (root == NULL)
	{
		return 0;
    }

	return BinaryTreeSize(root->left) + BinaryTreeSize(root->right) + 1;
}

⑥.二叉树的叶结点数

int BinaryTreeLeafSize(TreeNode* root)
{
	if (root == NULL)
	{
		return 0;
	}

	if (root->left==NULL&&root->right==NULL)
	{
		return 1;
	}

	int lefts= BinaryTreeLeafSize(root->left);
	int rights=BinaryTreeLeafSize(root->right);
	return lefts + rights;

	
}

⑦.二叉树的第K层结点数

int BinaryTreeLevelKSize(TreeNode* root,int k)
{
	if (root == NULL)
	{
		return 0;
	}

	if (k == 1)
	{
		return 1;
	}
	
	return BinaryTreeLevelKSize(root->left, k - 1)+ BinaryTreeLevelKSize(root->right, k - 1);
}

⑧.查找树为X的结点

TreeNode* BinaryTreeFind(TreeNode* root, char x)
{
	if (root == NULL)
	{
		return NULL;
	}

	if (root->data == x)
	{
		return root;
	}
	BinaryTreeFind(root->left,x);
	BinaryTreeFind(root->right,x);
}

⑨二叉树的层序遍历

TreeNode* BinaryTreeLevelOrder(TreeNode* root)
{
	if (root == NULL)
	{
		return NULL;
	}

	Queue q;
	QueueInit(&q);
	QueuePush(&q, root);

	while (!QueueEmpty(&q))
	{
		TreeNode* front = QueueFront(&q);
		QueuePop(&q);
		printf("%c", front->data);

		if (front->left)
			QueuePush(&q, front->left);
		if (front->right)
			QueuePush(&q, front->right);
	}
	QueueDestroy(&q);
	
}

 ⑩.判断完全二叉树

bool BinaryTreeComplete(TreeNode* root)
{
	Queue q;
	QueueInit(&q);
	//如果根存在，则进队列
	if(root)
	QueuePush(&q, root);

	//如果队列不为空
	while (!QueueEmpty(&q))
	{
		TreeNode* front = QueueFront(&q);
		QueuePop(&q);
		//如果front为空  跳出循环
		if (front==NULL)
		{
			break;   //进入二次判断
		}
		if (front->left)
		{
			QueuePush(&q, front->left);
		}
		if (front->right)
		{
			QueuePush(&q, front->right);
		}
	}

	//二次判断
	while (!QueueEmpty(&q))
	{
		TreeNode* front = QueueFront(&q);
		QueuePop(&q);

		if (front)
		{
			QueueDestroy(&q);
			return false;
		}
	}

	QueueDestroy(&q);
	return true;
}

 ⑪.二叉树的销毁

void BinaryTreeDestroy(TreeNode* root)
{
	if (root == NULL)
	{
		return NULL;
	}
	BinaryTreeDestroy(root->left);
	BinaryTreeDestroy(root->right);
	free(root);
}

四.完整代码 

1.queue.h

#include<stdio.h>
#include<stdlib.h>
#include<stdbool.h>
#include<assert.h>

typedef struct TreeNode* datatype;

typedef struct QNode
{
	datatype data;
	struct QNode* next;
}QNode;

typedef struct Queue
{
	QNode* head;
	QNode* tail;
	int size;
}Queue;

void QueueInit(Queue* pq);
void QueuePush(Queue* pq,datatype x);
void QueuePop(Queue* pq);
datatype QueueFront(Queue* pq);
datatype QueueBack(Queue* pq);
int QueueSize(Queue* pq);
bool QueueEmpty(Queue* pq);
void QueueDestroy(Queue* pq);

2.queue.c

void QueueInit(Queue* pq)
{
	assert(pq);

	pq->head = pq->tail = NULL;
	pq->size = 0;
}

void QueuePush(Queue* pq, datatype x)
{
	assert(pq);
	QNode* new = (QNode*)malloc(sizeof(QNode));
	if (new==NULL)
	{
	  perror("malloc fail\n");
	  return;
	}
	  new->data = x;
      new->next = NULL;

	if (pq->head == NULL)
	{
		assert(pq->tail == NULL);

		pq->head = new;
		pq->tail = new;
	}
	else
	{
		pq->tail->next = new;
		pq->tail = new;
	}

	pq->size++;


}
void QueuePop(Queue* pq)
{
	assert(pq);
	assert(pq->head != NULL);
	
	if (pq->head->next == NULL)
	{
		free(pq->head);
		pq->head = pq->tail = NULL;
	}
	else
	{
		QNode* next = pq->head->next;
		free(pq->head);
		pq->head = next;

	}

	pq->size--;

}
datatype QueueFront(Queue* pq)
{
	assert(pq);
	assert(!QueueEmpty(pq));

	return pq->head->data;
}
datatype QueueBack(Queue* pq)
{
	assert(pq);
	assert(!QueueEmpty(pq));

	return pq->tail->data;
}
int QueueSize(Queue* pq)
{
	assert(pq);

	return pq->size;
}
bool QueueEmpty(Queue* pq)
{
	assert(pq);

	if (pq->size == 0)
	{
		return true;
	}
	return false;
}

void QueueDestroy(Queue* pq)
{
	assert(pq);

	QNode* cur = pq->head;
	while (cur)
	{
		QNode*next=cur->next;
		free(cur);
		cur = next;
	}

	pq->head = pq->tail = NULL;
	pq->size = 0;
}

3.tree.c

typedef struct TreeNode
{
	char data;
	struct TreeNode* left;
	struct TreeNode* right;
}TreeNode;


TreeNode* rootBinaryTreeCreate(char* arr, int n, int* i)
{
	TreeNode* node = (TreeNode*)malloc(sizeof(TreeNode) * n);
	if (arr[*i] == '#')
	{
		(*i)++;              //++和* 优先级问题
		return NULL;
	}
	
	node->data = arr[(*i)++];            // ++和* 优先级问题
	node->left = rootBinaryTreeCreate(arr, n, i);
	node->right = rootBinaryTreeCreate(arr, n, i);

	return node;

}

void BinaryTreePrevOrder(TreeNode* root)
{
	if (root == NULL)
	{
		return NULL;
	}

	printf("%c ", root->data);
	BinaryTreePrevOrder(root->left);
	BinaryTreePrevOrder(root->right);
}


void BinaryTreeInOrder(TreeNode* root)
{
	if (root == NULL)
	{
		return NULL;
	}

	BinaryTreeInOrder(root->left);
	printf("%c ", root->data);
	BinaryTreeInOrder(root->right);
}

void BinaryTreePostOrder(TreeNode* root)
{
	if (root == NULL)
	{
		return NULL;
	}

	BinaryTreePostOrder(root->left);
	BinaryTreePostOrder(root->right);
	printf("%c ", root->data);
}

int BinaryTreeSize(TreeNode* root)
{
	if (root == NULL)
	{
		return 0;
    }

	return BinaryTreeSize(root->left) + BinaryTreeSize(root->right) + 1;
}

int BinaryTreeLeafSize(TreeNode* root)
{
	if (root == NULL)
	{
		return 0;
	}

	if (root->left==NULL&&root->right==NULL)
	{
		return 1;
	}

	int lefts= BinaryTreeLeafSize(root->left);
	int rights=BinaryTreeLeafSize(root->right);
	return lefts + rights;

	
}

int BinaryTreeLevelKSize(TreeNode* root,int k)
{
	if (root == NULL)
	{
		return 0;
	}

	if (k == 1)
	{
		return 1;
	}
	
	return BinaryTreeLevelKSize(root->left, k - 1)+ BinaryTreeLevelKSize(root->right, k - 1);
}

	TreeNode* BinaryTreeFind(TreeNode* root, char x)
	{
		if (root == NULL)
		{
			return NULL;
		}

		if (root->data == x)
		{
			return root;
		}
		BinaryTreeFind(root->left,x);
		BinaryTreeFind(root->right,x);
	}

TreeNode* BinaryTreeLevelOrder(TreeNode* root)
{
	if (root == NULL)
	{
		return NULL;
	}

	Queue q;
	QueueInit(&q);
	QueuePush(&q, root);

	while (!QueueEmpty(&q))
	{
		TreeNode* front = QueueFront(&q);
		QueuePop(&q);
		printf("%c", front->data);

		if (front->left)
			QueuePush(&q, front->left);
		if (front->right)
			QueuePush(&q, front->right);
	}
	QueueDestroy(&q);
	
}

bool BinaryTreeComplete(TreeNode* root)
{
	Queue q;
	QueueInit(&q);
	//如果根存在，则进队列
	if(root)
	QueuePush(&q, root);

	//如果队列不为空
	while (!QueueEmpty(&q))
	{
		TreeNode* front = QueueFront(&q);
		QueuePop(&q);
		//如果front为空  跳出循环
		if (front==NULL)
		{
			break;   //进入二次判断
		}
		if (front->left)
		{
			QueuePush(&q, front->left);
		}
		if (front->right)
		{
			QueuePush(&q, front->right);
		}
	}

	//二次判断
	while (!QueueEmpty(&q))
	{
		TreeNode* front = QueueFront(&q);
		QueuePop(&q);

		if (front)
		{
			QueueDestroy(&q);
			return false;
		}
	}

	QueueDestroy(&q);
	return true;
}

void BinaryTreeDestroy(TreeNode* root)
{
	if (root == NULL)
	{
		return NULL;
	}
	BinaryTreeDestroy(root->left);
	BinaryTreeDestroy(root->right);
	free(root);
}
int main()
{
	char arr[] = "ABD##E#H##CF##G##";
	int sz = strlen(arr);
	int i = 0;
	TreeNode* root=rootBinaryTreeCreate(arr,sz,&i);
	BinaryTreePrevOrder(root);       //先序遍历
	printf("\n");
	BinaryTreeInOrder(root);         //中序遍历
	printf("\n");
	BinaryTreePostOrder(root);       //后序遍历
	printf("\n");
	printf("二叉树的结点个数为:%d\n",BinaryTreeSize(root));        //二叉树的结点个数
	printf("二叉树的叶子结点个数为:%d\n",BinaryTreeLeafSize(root));   //二叉树的叶子结点个数
	printf("二叉树第k层结点个数为:%d\n", BinaryTreeLevelKSize(root,3));  //二叉树第k层结点个数
	printf("二叉树值为K的结点地址为:%p\n",BinaryTreeFind(root, 'C'));   //二叉树值为K的结点地址
	printf("二叉树的层序遍历:"); BinaryTreeLevelOrder(root);            //二叉树的层序遍历
	printf("\n判断二叉树是否为完全二叉树:%d\n",BinaryTreeComplete(root)); //判断二叉树是否为完全二叉树
	BinaryTreeDestroy(root);
	root = NULL;

}

JJY_s
关注
评论
添加红包

请填写红包祝福语或标题

个

红包个数最小为10个

元

红包金额最低5元

当前余额3.43元 前往充值 >
需支付：10.00元
成就一亿技术人!
领取后你会自动成为博主和红包主的粉丝 规则
hope_wisdom
发出的红包

打赏作者

JJY_s

你的鼓励将是我创作的最大动力

¥1 ¥2 ¥4 ¥6 ¥10 ¥20
扫码支付：¥1
获取中
扫码支付

您的余额不足，请更换扫码支付或充值

打赏作者

实付元
使用余额支付
点击重新获取
扫码支付
钱包余额 0

抵扣说明：

1.余额是钱包充值的虚拟货币，按照1:1的比例进行支付金额的抵扣。
2.余额无法直接购买下载，可以购买VIP、付费专栏及课程。

余额充值