CodeForces 510B Fox And Two Dots

最新推荐文章于 2019-07-24 17:14:33 发布

原创最新推荐文章于 2019-07-24 17:14:33 发布 · 562 阅读

0 ·

CC 4.0 BY-SA版权

2015 Winter Camp 专栏收录该内容

11 篇文章

订阅专栏

本文介绍了一种使用深度优先搜索(DFS)解决二维网格中寻找相同颜色点构成的环形路径的方法。通过方向标记避免了深度为2时的误判。

Fox Ciel is playing a mobile puzzle game called "Two Dots". The basic levels are played on a board of size n × m cells, like this:

$\text{[math]}$

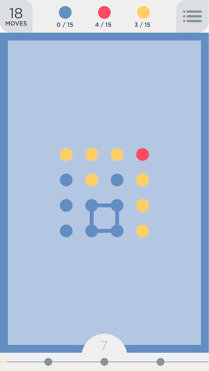
Each cell contains a dot that has some color. We will use different uppercase Latin characters to express different colors.

The key of this game is to find a cycle that contain dots of same color. Consider 4 blue dots on the picture forming a circle as an example. Formally, we call a sequence of dots d1, d2, ..., dk a cycle if and only if it meets the following condition:

These k dots are different: if i ≠ j then di is different from dj.
k is at least 4.
All dots belong to the same color.
For all 1 ≤ i ≤ k - 1: di and di + 1 are adjacent. Also, dk and d1 should also be adjacent. Cells x and y are called adjacent if they share an edge.

Determine if there exists a cycle on the field.

Input

The first line contains two integers n and m (2 ≤ n, m ≤ 50): the number of rows and columns of the board.

Then n lines follow, each line contains a string consisting of m characters, expressing colors of dots in each line. Each character is an uppercase Latin letter.

Output

Output "Yes" if there exists a cycle, and "No" otherwise.

Sample test(s)

input
3 4
AAAA
ABCA
AAAA

output
Yes

input
3 4
AAAA
ABCA
AADA

output
No

input
4 4
YYYR
BYBY
BBBY
BBBY

output
Yes

input
7 6
AAAAAB
ABBBAB
ABAAAB
ABABBB
ABAAAB
ABBBAB
AAAAAB

output
Yes

input
2 13
ABCDEFGHIJKLM
NOPQRSTUVWXYZ

output
No

Note

In first sample test all 'A' form a cycle.

In second sample there is no such cycle.

The third sample is displayed on the picture above ('Y' = Yellow, 'B' = Blue, 'R' = Red).

这道题很容易理解，就是判断是否存在相同颜色的小球构成环

大致思路就是用DFS，直到找到已经标记过的点，那么就找到一个环了

但是同时还要考虑到搜索深度的问题，因为当深度为2时，根据判断条件会误判为已经找到满足题意环

训练赛的时候一直想不好该怎么处理，快结束的时候就放弃欢脱地去吃饭了~(≧▽≦)/~

饭饱茶足回来，查了下别人的做法，真的很精练啊

改成了自己的代码交了上去

#include<iostream>
#include<cstring>
using namespace std;

const int maxn = 50+5;

char G[maxn][maxn];
bool idx[maxn][maxn] = {false};

bool dfs(int i, int j, int t) {
  if (idx[i][j] == true) return true;
  idx[i][j] = true;
  if (G[i][j] == G[i-1][j] && t!=2) if (dfs(i-1,j,1)) return true;
  if (G[i][j] == G[i+1][j] && t!=1) if (dfs(i+1,j,2)) return true;
  if (G[i][j] == G[i][j-1] && t!=4) if (dfs(i,j-1,3)) return true;
  if (G[i][j] == G[i][j+1] && t!=3) if (dfs(i,j+1,4)) return true;
  return false;
}

int main() {
  int n, m;
  cin >> n >> m;
  memset(G, 0, sizeof(G));
  for (int i = 0; i < n; i++)
    cin >> G[i];
  for (int i = 0; i < n; i++)
    for (int j = 0; j < m; j++)
      if (!idx[i][j])
        if (dfs(i, j, 0)) {
          cout << "Yes" << endl;
          return 0;
        }
  cout << "No" << endl;
  return 0;
}

dfs函数还是很常见的写法，唯一值得一提的是增加了一个参量t用来记录搜索时的方向

这样就可以避免误判了，真是机智(^o^)/